The heat of hydrogenation of ethene is #x_1# and that of Benzene is #x_2#. Hence resonance energy is?

I got #x_2 - 3x_1#, defining #DeltaE_"reso"# with benzene being the final state.

So, none of the above, apparently.

From experimental data, we have:

#3DeltaH_(hyd)("C"_2"H"_4) = 3(-"137.37 kJ/mol") -= 3x_1#
(http://nvlpubs.nist.gov/nistpubs/jres/17/jresv17n5p629_A1b.pdf, pg. 5)

#DeltaH_(hyd)("benzene") = -"205.43 kJ/mol" -= x_2#
(http://pubs.acs.org/doi/pdf/10.1021/ja001274p)

But this doesn't make any sense. It neglects the formation of bonds among the ethenes, which would make cyclohexatriene more stable and harder to hydrogenate than three ethenes.

That means there is less energy benefit from hydrogenating cyclohexatriene than three ethenes, and so,

#3DeltaH_(hyd)("C"_2"H"_4) < DeltaH_(hyd)("cyclohexatriene")#.

Actually,

#DeltaH_(hyd)("cyclohexatriene") = -"354.8 kJ/mol"#
(http://pubs.acs.org/doi/pdf/10.1021/ja001274p)

#3DeltaH_(hyd)("C"_2"H"_4) = -"412.11 kJ/mol"#
(multiply out from the above)

So actually,

#color(red)(DeltaE_"reso"("unreasonable")) = overbrace((-"205.43 kJ/mol"))^(x_2) - overbrace(-"412.11 kJ/mol")^(3x_1 ("crude approx."))#

#=# #color(red)("206.68 kJ/mol")#, or #"49.4 kcal/mol"#

#color(blue)(DeltaE_"reso") = overbrace((-"205.43 kJ/mol"))^(x_2) - overbrace(-"354.8 kJ/mol")^(3x_1 ("corrected"))#

#=# #color(blue)("149.37 kJ/mol")#, or #"35.7 kcal/mol"# (as stated here).

A crude approximation is that the heat of hydrogenating three ethenes is approximately equal to hydrogenating cyclohexatriene.

Obviously that is not true. They are not connected in a ring... so this is a badly constructed problem.

(That is also not what benzene is, but that is not what is poorly-conceived about this problem.)

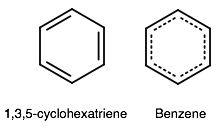
Benzene delocalizes its #pi# electrons, and from doing that it has a lower energy than cyclohexatriene. In general,

#DeltaE_"reso" = E_"benzene" - E_"cht"#,

the final delocalized state minus the initial localized state.

But we now revise this in terms of heat of hydrogenation. The heat of hydrogenation, a negative quantity, for this problem, asks for:

#3"C"_2"H"_4(g) + 3"H"_2(g) stackrel("High P ")(->) 3"C"_2"H"_6(g)#,

#3DeltaH_(hyd) -= 3x_1#

#"C"_6"H"_6(l) + 3"H"_2(g) stackrel("High P ")(->) "C"_6"H"_12(l)#,

#DeltaH_(hyd) -= x_2#

Since cyclohexatriene is less stable than it would be if it had resonance, it ought to release more energy to hydrogenate it than it would to hydrogenate benzene in order to compensate for it being less stable than the resonated case.

So we expect that #|3x_1| > |x_2|#, but that #3x_1# is more negative.

I defined resonance energy to be benzene as the final state, so to get that, it must be that

#color(blue)(DeltaE_"reso" = x_2 - 3x_1) > 0#,

So it's none of the above.