How to determine the temperature change for dissolving #"10.0 g"# of #"KClO"_4(s)# into water?

A practice problem in my textbook says...
"Potassium perchlorate has a lattice energy of -599 kJ/mol and a heat of hydration of -548 kJ/mol.
Find the heat of solution and determine the temperature change that happens when 10.0g of potassium perchlorate is dissolved with enough water to make 100.0 mL of solution.
(heat capacity of solution=4.05 J/g*C, density of solution= 1.05 g/mol."

I found the heat of solution as 51 kJ/mol, but I'm not sure where to go from here. I got 12.2 Celsius but that's not the answer. Thanks in advance!

1 Answer
Truong-Son N.
Oct 1, 2017

The solution cooled by #8.66^@ "C"#.

I would construct a thermodynamic cycle like this:

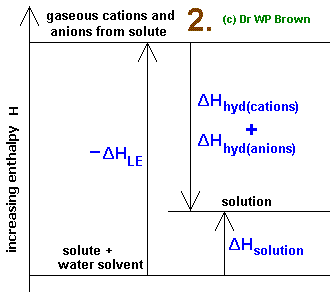
http://www.docbrown.info/

Here up is positive and down is negative, so:

#-DeltaH_L = +"599 kJ/mol"#
#DeltaH_"hyd" = -"548 kJ/mol"#

with #DeltaH_"soln" > 0#. And you should in fact get

#"51 kJ/mol KClO"_4(s)#

to turn #"1 mol"# of #"KClO"_4(s)# into #"K"^(+)(aq)# and #"ClO"_4^(-)(aq)#, an endothermic process.

When some amount of #"KClO"_4(s)# dissolves into water, it therefore absorbs heat from the water since #DeltaH_"soln"# is endothermic with respect to the solute particles (#"K"^(+)(aq)# and #"ClO"_4^(-)(aq)#).

Hence, we treat the solution as the surroundings and the ions as the system.

Given the solution density (not in #"g/mol"#, but what units?), we can then find the mass of the solution we actually have:

#100.0 cancel"mL soln" xx "1.05 g"/cancel"mL" = "105.0 g soln"#

Since #"51 kJ"# of heat is absorbed from the water due to dissolving #"1 mol"# of #"KClO"_4(s)#, and heat is an extensive quantity, we can find the heat absorbed from the water due to dissolving #"10.0 g KClO"_4(s)#:

#"51 kJ"/(cancel("1 mol KClO"_4) xx "138.55 g KClO"_4/cancel"1 mol") = (q_"solute"" kJ")/("10.0 g KClO"_4)#

#q_"solute" = "3.681 kJ"# absorbed by the solute from the surrounding solution.

By conservation of energy,

#q_"solute" + q_"solvent" ~~ 0#,

so #q_"solvent" = -"3.681 kJ"# describes the flow of #"3681 J"# of heat out of the water due to forming the ions.

Given the specific heat capacity of the solution and knowing its mass from earlier, the heat involved in changing the temperature of the solution is:

#q_"solvent" ~~ m_"soln"C_(P,"solvent")DeltaT#

And so, we can plug in what we know to solve for the change in temperature:

#-"3681 J" = "105.0 g soln" xx "4.05 J/g"^@ "C" xx DeltaT#

#=> color(blue)ul(DeltaT = -8.66^@ "C")#

And so the solution cooled, as we would expect from an endothermic process (such as ice packs).

Related questions
Impact of this question
1970 views around the world
You can reuse this answer
Creative Commons License