Consider the molecule [MnX6]2+, where X is a neutral ligand. Assume that X is a strong field ligand?

DISCLAIMER: Long answer!

#A)#

THE NUMBER OF 3D VALENCE ELECTRONS IN THE COMPLEX

When you examine the location of #"Mn"# on the periodic table, you should find that the electron configuration ends in #4s^2 3d^5#.

Since #"X"# is stated to be a neutral ligand, #"Mn"# in #["MnX"_6]^(2+)# by charge conservation is a #+2# oxidation state. Therefore, we doubly ionize neutral manganese to obtain the electron configuration #3d^5#, removing the two #4s# electrons first.

HIGH-SPIN VS LOW-SPIN, AS IT RELATES TO STRONG-FIELD AND WEAK-FIELD LIGANDS

A #d^5# metal such as this can form either a high-spin or low-spin complex, depending on the crystal field strength of the ligand.

We assume strong-field for #"X"#, which means that it can either be:

• A #sigma# donor (such as #:"NH"_3#, #"CO"#, or #""^(-)"CN"#)
• A #pi# acceptor (such as #"CO"# or #""^(-)"CN"#)
• Both (such as #"CO"# or #""^(-)"CN"#)

SIGMA DONORS

Let's say we just have a good #sigma# donor.

In this case, it destabilizes the #e_(g)# orbitals (there are two of those orbitals), since those interact with the #sigma# bonding molecular orbitals brought in by the #sigma# donor(s). #"CO"# is an example:

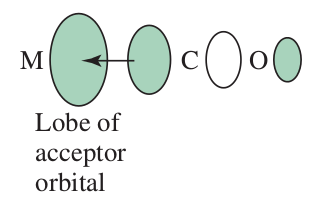
As a result, the two upper-energy (#e_g#) orbitals get higher in energy (relative to the free-ion energy), increasing the crystal-field splitting energy #Delta_o# (#Delta# in your diagram), promoting low spin.

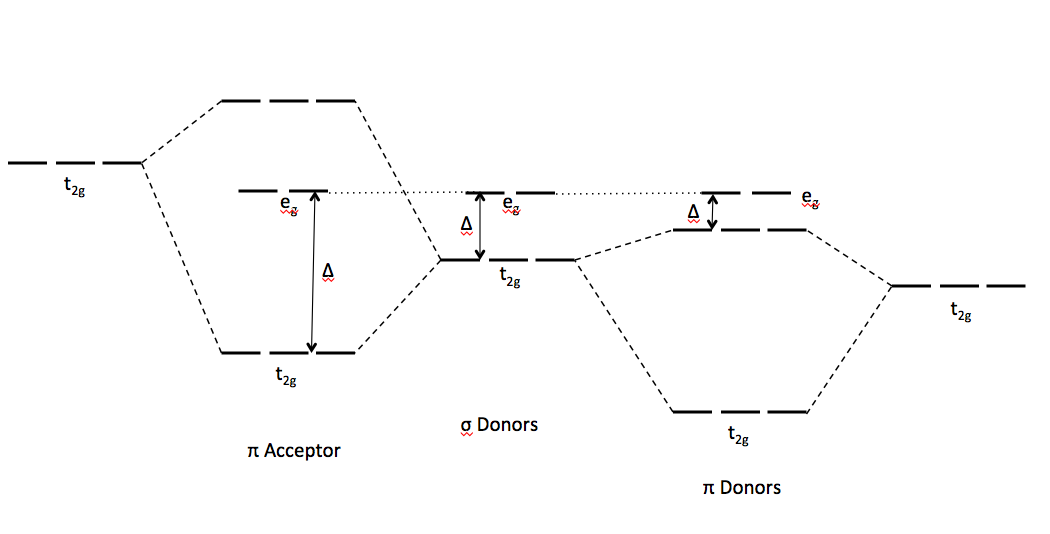
PI ACCEPTORS

Or, let's say we have a good #pi# acceptor.

In this case, the #pi# antibonding molecular orbitals of the ligand accept electrons from the #3# lower-energy (#t_(2g)#) #3d# orbitals.

This stabilizes these #3# lower-energy (#t_(2g)#) #3d# orbitals, decreasing their energy. This also increases #Delta_o#.

Since #"CO"# is both, it is one of the strongest-field ligands there is.

CRYSTAL-FIELD SPLITTING DIAGRAM

When a transition metal complex has a coordination number of #6# (given that it has six #"X"# ligands bound to it), it forms an octahedral electron geometry.

The diagram you were given is the crystal-field splitting diagram for the five #3d# orbitals of manganese once the manganese(II) complex is placed into an octahedral field that splits its #d#-orbital energies.

Here is the filled diagram for a manganese(II) complex in high spin or low spin:

The difference is that for a low spin complex, which corresponds to having a strong-field ligand, #Delta_o# is large.

So the electrons can capably fill the #3# lower-energy #t_(2g)# orbitals first... before they can feasibly fill the #2# higher-energy #e_g# orbitals.

#B)#

PI DONORS

A weak-field ligand contributes little repulsion. Actually, weak-field ligands are typically #pi# donors, and weak #sigma# donors.

If you have a #pi# donor, such as any halide (#"Cl"^(-)#, #"F"^(-)#, etc):

It has filled #pi# bonding molecular orbitals that donate into the three (#t_(2g)#) #3d# orbitals and destabilize them, increasing their energy.

That actually decreases #Delta_o#, and promotes high spin.

So, #Delta_o# would be smaller for a weaker-field ligand.

This diagram summarizes the trends for #pi# acceptors, #sigma# donors, and #pi# donors:

#C)#

CRYSTAL-FIELD SPLITTING ENERGY

Since the light absorbed will promote electrons from the #t_(2g)# into the #e_g# orbitals, the size of #Delta_o# tells you how much energy this light frequency corresponds to.

Since #Delta_o# becomes smaller when switching to a weaker-field ligand, and #Delta_o prop E prop nu#, a smaller frequency of light can be absorbed than before.

(Thus, you can also say that a longer wavelength is absorbed.)