Can we treat stretch mode in vibrational analysis as Harmonic oscillator?

Sometimes, but it would usually be a crude approximation if too many atoms are bundled into one "effective atom" on either side of the oscillator, i.e. if you did benzene, then it wouldn't make much sense to treat one #"H"# as one atom and #"C"_6"H"_5# as the other "atom".

For example, suppose we took methane and used the frequency of its #T_2# stretching mode:

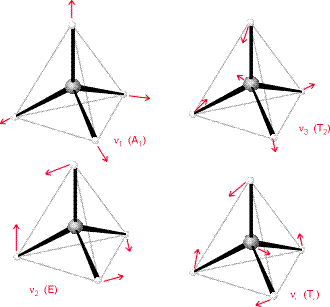
Let's pretend that the two hydrogens are one effective atom on each side, so that the reduced mass of each side is:

#mu = (m_1m_2)/(m_1 + m_2) = m_H^2/(2m_H) = m_H/2#

#= "0.0010079 kg/2"/"mol" xx ("1 mol")/(6.0221413 xx 10^23)#

#= 8.368 xx 10^(-28) "kg"#

And the collective reduced mass #mu'# used in the simple harmonic oscillator model would then be:

#mu' = (mu^2)/(2mu) = mu/2 = 4.184 xx 10^(-28) "kg"#

We can then check if this is a reasonable approximation by using the actual vibrational frequency of #"3104 cm"^(-1)# calculate a hypothetical force constant by using #mu'# and #"3104 cm"^(-1)# for the vibrational frequency #tildeomega_e#.

We don't expect this to give a good value.

#tildeomega_e = 1/(2pic) sqrt(k/(mu'))#

#=> k = 4pi^2c^2 tildeomega_e^2mu#

#= 4pi^2 cdot (2.998 xx 10^(10) "cm/s")^2("3104 cm"^(-1))^2(4.184 xx 10^(-28) "kg")#

#= "143.05 kg/s"^2#

The typical force constant of a bond in a diatomic molecule is a few hundred to a few thousand #"kg/s"^2#, so this makes SOME physical sense.

However, this is unusually small, as it would suggest a weaker "bond" than #"HI"# (with #k = "320 kg/s"^2#), so this approximation is not that great. In essence, it had required that we treat a four-atom pivot point as a chemical bond, which is a hard sell.

This is not usually done in practice, and this value has not been tabulated anywhere because it is not very useful.