Answer key for a chemistry table (chapter 12)?
I have a chemistry table from chapter 12 of some book somewhere and I need to find an answer key so I can figure out how to do the problems on it.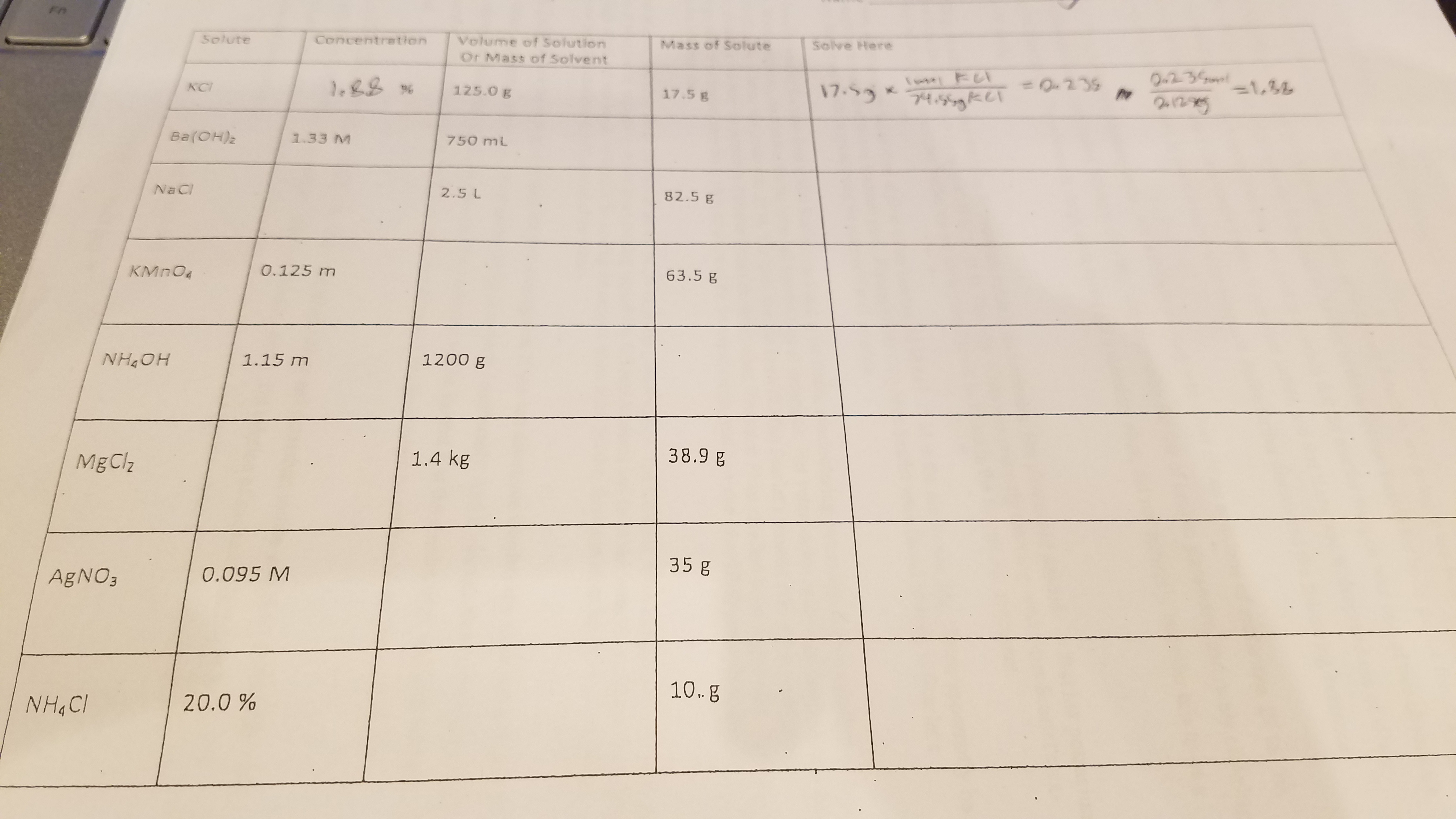
I have a chemistry table from chapter 12 of some book somewhere and I need to find an answer key so I can figure out how to do the problems on it.
1 Answer
Well, I can provide three of them, but you'll have to work the rest out yourself.
For
For
For
For
General definitions you MUST know...
#"Molarity" = "mol solute"/"L solution"#
#"Molality" = "mol solute"/"kg solvent"#
#%"w/w" = "g solute"/"100 g solution"#
#"mass solution = mass solute + mass solvent"#
And of course,
#"1000 g"# #=# #"1 kg"#
#"1000 mL"# #=# #"1 L"#
Taking the
#1200 cancel"g solvent" xx cancel"1 kg"/(1000 cancel"g") xx "1.15 mol solute"/cancel"kg solvent"#
#= 1.3_8# #"mols solute"#
where the subscript indicates the digit past the last significant digit. And thus, knowing the molar mass, the mass of ammonium hydroxide found in this solution is...
#1.3_8 cancel("mols NH"_4"OH") xx "35.046 g"/cancel("1 mol NH"_4"OH") = color(blue)("48 g NH"_4"OH")#
(with the solution presumed aqueous ammonia, if water is the solvent), to two sig figs.
Given
#38.9 cancel("g MgCl"_2) xx "1 mol"/(95.211 cancel("g MgCl"_2)) = "0.408"_6# #"mols"#
And so, the molality of the solution is:
#(0.408_6 "mols solute")/("1.4 kg solvent") = color(blue)("0.29 mol/kg")#
or simply
From "
#=> "20. g solute"/"100 g soln" = "10. g solute"/"50 g soln"# (where the 100 and 50 g are exact!)
We know that in terms of mass, solution = solute + solvent... so the mass of the solvent is...
#"50 g soln" - "10. g solute" = color(blue)("40. g solvent")#

