According to the molecular orbital theory, what is the bond order in #B_2^+#?

#"BO" = 1/2#

Boron atom is atomic number #5# in the periodic table, so it has five electrons. Thus, #B_2# carries ten total electrons. The atomic orbitals each boron contributes consists of the #1s#, #2s#, and #2p#.

The #ns# orbitals combine to give a portion of the molecular orbital (MO) diagram like this:

where #sigma^"*"# indicates an antibonding #sigma# (sigma) MO, and #sigma# is the bonding MO.

The antibonding MO is higher in energy because it has one more node than the corresponding bonding MO, and thus, the electrons are closer together (having less room to be), and repel each other more (increasing the destabilizing electron repulsion energy).

Analogous atomic orbital combinations for the #np_z# and #np_(x"/"y)#, which are significantly collectively higher in energy than the #sigma_(ns)# and #sigma_(ns)^"*"# MOs, give:

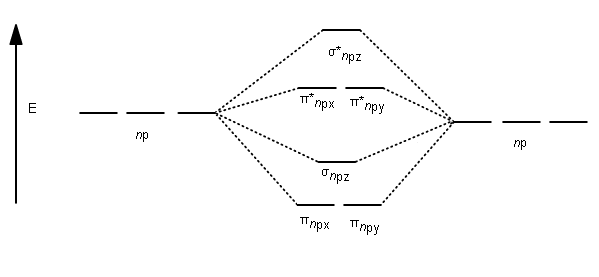
For the homonuclear diatomic molecules #Li_2# through and including #N_2#, the orbital energy ordering is as it is above. At #O_2# and past, the ordering of the #sigma_(2p_z)# and #pi_(2p_(x"/"y))# switch.

Filling the molecular orbitals:

• The first four electrons fill the #sigma_(1s)# and #sigma_(1s)^"*"# MOs.
• The second four electrons fill the #sigma_(2s)# and #sigma_(2s)^"*"# MOs.
• The last two electrons singly occupy the #pi_(2p_x)# and #pi_(2p_y)# MOs.

The bond order for #B_2# would be:

#"BO" = 1/2("Bonding electrons - Antibonding electrons")#

#= 1/2[(2 + 2 + 1 + 1) - (2 + 2)]#

#= 1/2(2) = 1#

That is, we expect boron to form this compound with itself:

#:"B"-"B":#

However, since you asked for #B_2^+#, we remove one bonding #pi_(2p_(y))# electron, decreasing the bond order by #1/2#.

So, #color(blue)("BO")# #=# #color(blue)(1/2)# for #B_2^+#. What can you say about the bond strength and length relative to those for #B_2#?