Question #91ae7

Well, the Bohr model ONLY works for the hydrogen atom, so this is only useful for the hydrogen atom... or atoms with one electron (which are not that useful).

For multi-electron atoms, we must account for electron correlation, which splits the orbitals by their angular momentum (e.g. #3s, 3p, 3d#, not just "#3#").

Clearly, a given #n# will have multiple energy sublevels (#s,p,d,f,g, ... #), and it is insufficient to only have one quantum number #n# to describe the energies.

We know the Rydberg equation

#DeltaE = E_(n_f) - E_(n_i) = -"13.6 eV"(1/n_f^2 - 1/n_i^2)#,

which is really just using initial and final states #E_(n_i)# and #E_(n_f)# for an electron's energy transition in the hydrogen atom from initial to final principal quantum number, #n_i# to #n_f#.

So, taking only one of these states gives

#bb(E_n = -(13.6 "eV")/(n^2))#

for the energy level for the principal quantum number #n# in the HYDROGEN atom.

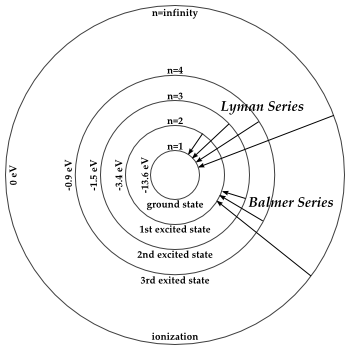
You can see this work for the hydrogen atom.

#E_2 = -"13.6 eV"/(2^2) = -"3.4 eV"#

#E_3 = -"13.6 eV"/(3^2) = -"1.51 eV" ~~ -"1.5 eV"#

#E_4 = -"13.6 eV"/(4^2) = -"0.85 eV" ~~ -"0.9 eV"#

etc.